BTU auf einen Blick
Fakultät 3
Lehrstuhl Aerodynamik und Strömungslehre Prof. Dr.-Ing. Christoph Egbers

Space Applications

TEKUS

Thermoelectric convection in weightlessness (DLR FKZ: 50WM1944)

We study the influence of the dielectrophoretic (DEP) effect inducing electrically driven buoyancy in microgravity conditions in order to separate this effect from Archimedean buoyancy. In microgravity conditions we can pose the question, how efficiently "electric gravity" can replace the gravitational effect. After the execution of a series of parabolic flight experiment campaigns we are preparing now sounding rocket flight experiments within the TEXUS-program of the German Space Agency.

Subsequently, we performed parabolic flight campaigns (PFCs) supported by the German Aerospace Center DLR, "14th DLR PFC" (September 2009; Testflight), "16th DLR PFC" (November 2010), "19th DLR PFC" (February 2012), 22nd DLR PFC (April 2013), 25th DLR PFC (Octtober 2014), 31st DLR PFC (September 2017),  34th  DLR PFC (March 2018) and also three PFC (118th , 134th and 139th) supported by Centre Nationale d’Études Spatiales (CNES) as a cooperative project with LOMC, Normandie University, Le Havre, France in October 2015, 2016 and 2018.

The application of the dielectrophoretic force under low-g and 1g conditions showed that the flow becomes unstable when the electric tension is sufficiently large. The shape of the unstable regimes depends on the aspect ratio of the cylindrical annulus, on the gravitational condition and on the set of control parameters. Under low-g conditions, the preferred critical mode is mainly helical, at least for low to moderate temperature differences between the two cylinders.

Both gravitational levels have been studied through a linear stability theory (Yoshikawa et al. 2013, Meyer et al. 2017) as well as through numerical simulations (Travnikov et al.) and exhibit a qualitatively good agreement with our experimental results. However, the threshold for the occurrence of instabilities is at larger electric potential in the experiments. The top and bottom boundaries stabilize the flow, and it is clear that for sufficiently large temperature differences, the Boussinesq approximation is no more valid.

Theoretical researches have considered microgravity conditions in order to focus on the DEP force, see e.g. Takashima 1979, but laboratory experiments have to involve the Earth’s gravity effect, which is modifying the stability conditions of the system (see Seelig et al. 2019). Theoretical and numerical research presents an analogy between both convective modes (see B. Chandra and D. E. Smylie, 1972; S. Malik, et al. 2012; H. N. Yoshikawa et al., 2013 a); H. N. Yoshikawa et al., 2013 b)). B. Sitte and H.J. Rath (2003) verified the DEP-induced buoyancy effect by laboratory and parabolic flight experiments. 3-D numerical simulations have been performed by Travnikov et al. 2013, Kang and Mutabazi 2019 and Gerstner (see Seelig et al. 2019).

Using PIV and Shadowgraph methods, it has been found that, under Earth’s gravity condition, the first unstable regime takes the form of stationary columnar counter-rotating vortices. A stability diagram spanned by the thermal Rayleigh number and the dimensionless electric potential has been built experimentally and compared to results from a linear stability analysis (see Meyer et al. 2017). For a set of given control parameters, the experimental measurements of velocity and temperature have been compared to numerical simulations and showed a good qualitative agreement. Indeed the columnar structure has been confirmed experimentally, numerically and theoretically and could be explained by simultaneous effect of the axial Earth gravity and the radial electric gravity.

The low gravity phase provided by the parabolic flights lasts about 22s, which is too short to etablish a fully developed thermoelectric instability in our experimental parameter range. But, it has been possible to detect the growth of perturbations which allowed us to build a stability diagram, to characterize the geometry of the flow, and to compare the growth rates of perturbations between Earth’s gravity and weightlessness environment.

Consequently, we are now preparing a sounding rocket flight experiment - foreseen for the TEXUS 57 campaign. For this experimental set-up, we developed a combination of Shadowgraph and PIV-techniques which we apply simultaneously for each experiment cell. Figure 1 gives an example for the processed flow field images of the cylindrical gap for natural and DEP-forced convection under earth conditions. The combination of the two measurement techniques allows the simultaneous visualization of the flow pattern in vertical (integrated over the height) and meridional direction.

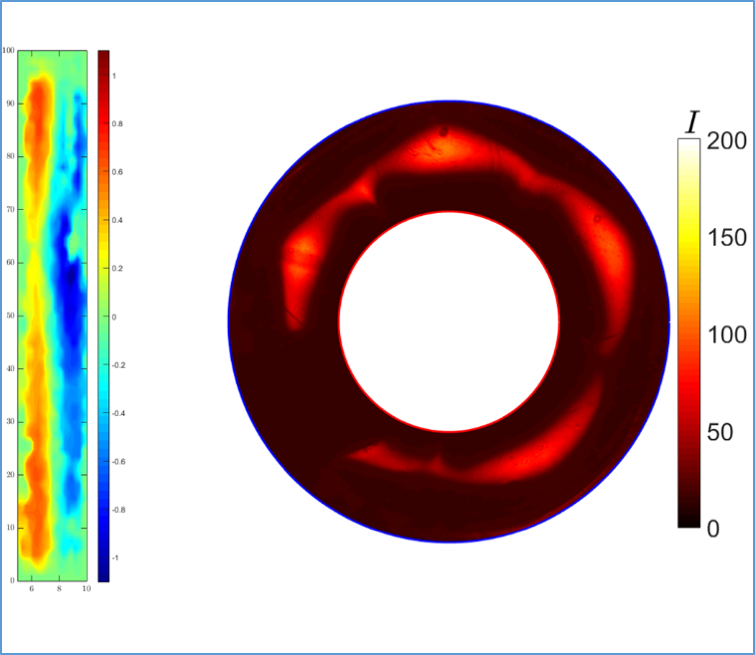
Fig. 1: Simultaneous measurement of the flow field inside the cylindrical gap. Experiment parameters: ΔT=2K, T0=25°C, 1g. Natural convection with basic flow. Left picture from PIV-analysis; right picture Shadowgraph image.

By using the TEXUS-rocket with 6 minutes of microgravity time we will be able to determine the flow stability, the mode shape and the corresponding heat transfer in our TEHD-experiments with much higher accuracy than in parabolic flights.

References

  1. B. Chandra, D. E. Smylie, A laboratory model of thermal convection under a central force field. Geophysical Fluid Dynamics, 3 (1972) 211- 224.
  2. B. Futterer, N. Dahley, C. Egbers, Thermal electro-hydrodynamic heat transfer augmentation in vertical annuli by the use of dielectrophoretic forces through a.c. electric field. Int. J. Heat Mass Transfer 93 (2016).
  3. C. Kang, I. Mutabazi, Dielectrophoretic buoyancy and heat transfer in a dielectric liquid contained in a cylindrical annular cavity, J. App. Phys. 125 (2019), doi.10.1063/1.5086980.
  4. S. Malik, H.N. Yoshikawa, O. Crumeyrolle, I. Mutabazi, Thermo-electro-hydrodynamic instabilities in a dielectric liquid under microgravity. Acta Astronautica 81 (2012) 563-569.
  5. M. Meier, M. Jongmanns, A. Meyer, T. Seelig, C. Egbers, I. Mutabazi, Flow pattern and heat transfer in a cylindrical annulus under 1g and low-g conditions:  Experiments, Microgravity Sci. Technol. 30 (2018) 699-712.
  6. A. Meyer, M. Jongmanns, M. Meier, C. Egbers, I.Mutabazi, Thermal convection in a cylindrical annulus under a combined effect of the radial and vertical gravity, C. R. Mecanique 345 (2017) 11–20.
  7. A. Meyer, Crumeyrolle, O., Mutabazi, I., Meier, M., Jongmanns, M., Renoult, M.-C., Seelig, T., Egbers, C.: Flow Patterns and Heat Transfer in a Cylindrical Annulus under 1g and low-g Conditions: Theory and Simulation, Microgravity Sci. Technol. 30 (2018) 653–662.
  8. T. Seelig, A. Meyer, P. Gerstner, M. Meier, M. Jongmanns, M. Baumann, V. Heuveline, C. Egbers, Dielectrophoretic force-driven convection in annular geometry under Earth’s gravity, International Journal of Heat and Mass Transfer (accepted in April 2019)
  9. B. Sitte, H. J. Rath, Influence of the dielectrophoretic force on thermal convection. Exp. Fluids (2003) vol. 34, pp. 24-27.
  10. Travnikov, O. Crumeyrolle, I. Mutabazi, Numerical investigation of the heat transfer in cylindrical annulus with a dielectric  fluid under microgravity. Phys. Fluids, vol. 27 (2015) 054103.
  11. M. Takashima, “Electrohydrodynamic instability in a dielectric fluid between two coaxial cylinders”, Mech. appl. Math. 33 (1979) 93-103. 
  12. H.N. Yoshikawa, O. Crumeyrolle, I. Mutabazi, Dielectrophoretic force-driven thermal convection in annular geometry. Phys. Fluids, vol. 25 (2013) 024106